Having just made the chestahedron template, I thought I’d make a few and see how it went.  I noticed that six chestahedra fit perfectly together in a star pattern:

Although the form seems to fit flush with the table surface, it actually forms a concave curve, because the six chestahedra angle outward from the center point.  This made me wonder: “What is that curve? What if it could be extended?”

I made a few more chestahedra and quickly saw that indeed the curve could be continued quite easily.  It was apparent, because of the nature of the equilateral triangular base of the chestahedron, that a three-fold symmetry was at work.  The concave surface was made of six triangles that formed a concave hexagon, and the curve could be continued in any direction.  I wanted to know if the curve, which obviously formed a circle, would actually meet itself in a way that all the forms fit perfectly together.  Perhaps the curve necessitated by the hexagonal tiling was not able to be closed with an integer number of chestahedra.

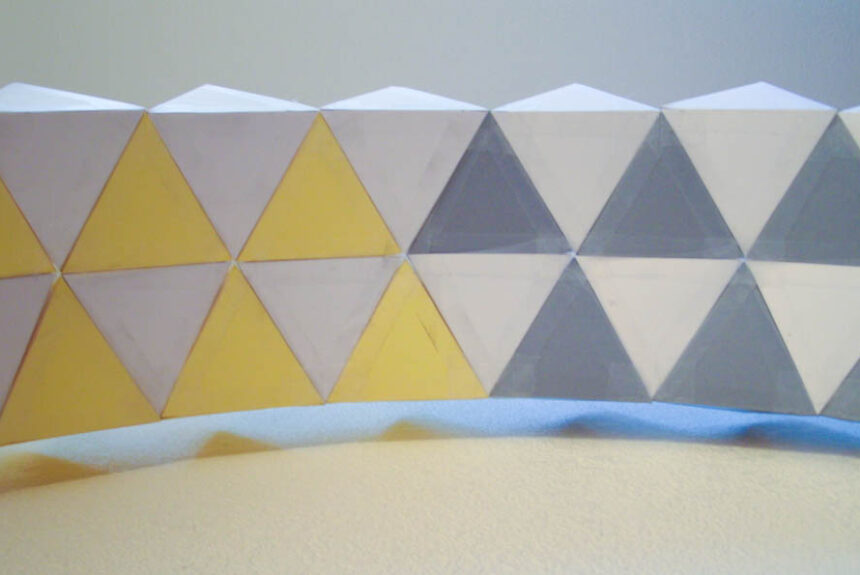
I did not know how many chestahedra would be needed to test this theory, but based on the curve formed by six together it seemed like it wouldn’t be so many as to be impractical.  It turns out that 96 chestahedra are needed to form what I call the chestahedral ring.

The form was very beautiful and sculpturally intriguing.  The curve of the ring is necessitated by the way the individual chestahedra interlock; there is only one chestahedral ring when configured in this way.  The forms are made out of #60 card stock and scotch tape.  The equilateral triangles have a side length of approximately three inches, and this makes the final inner diameter of the form approximately 22 inches.

Obviously the form could have extended in any other direction; it seemed that in fact that this particular tiling suggested a complete sphere, the chestahedral sphere.  Triangles easily tile the surface of a sphere, as Buckminster Fuller discovered with the geodesic domes.  The chestahedral sphere is a form of geodesic dome on the inside, but what is unique about this form is its three-dimensionality.  Each of the three side triangles on an individual chestahedra adjoins others from neighboring chestahedra, giving the whole form a particular necessary self-integration that works with the full volume of space.  Contrast this with the famous geodesic sphere of the Epcot Center, where the triangular elements of the dome are simply raised at their center point into flattened tetrahedra, which have no structural relevance.  The chestahedral sphere is integrated structurally in three dimensions. This all flows naturally and necessarily from the specific form of the chestahedron itself.  The chestahedron, which is made only of straight lines, still has as a part of its nature a curve.

It was obvious that I would have to make more chestahedra… Another 96 chestahedra later, (actually fewer are needed because part of the ring is already built – if you are landing on this page, visit the background for this form here), and the following form appeared:

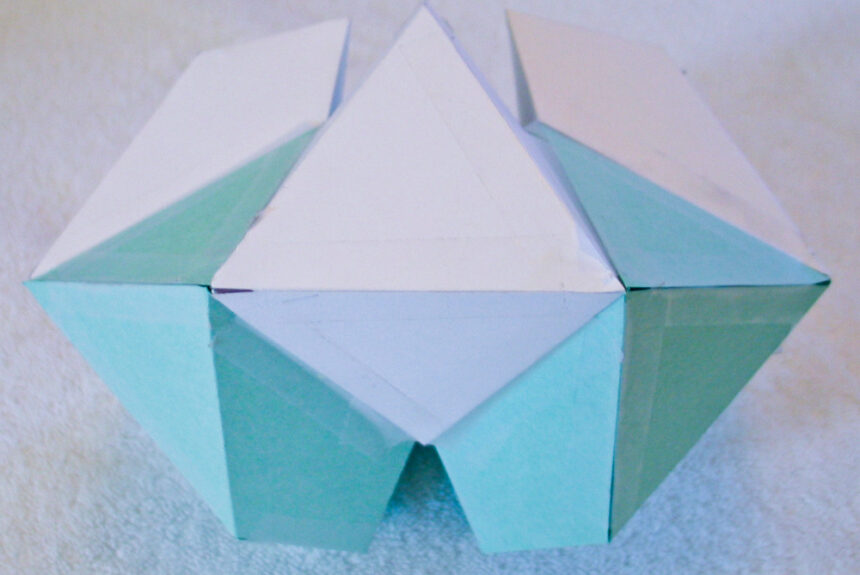
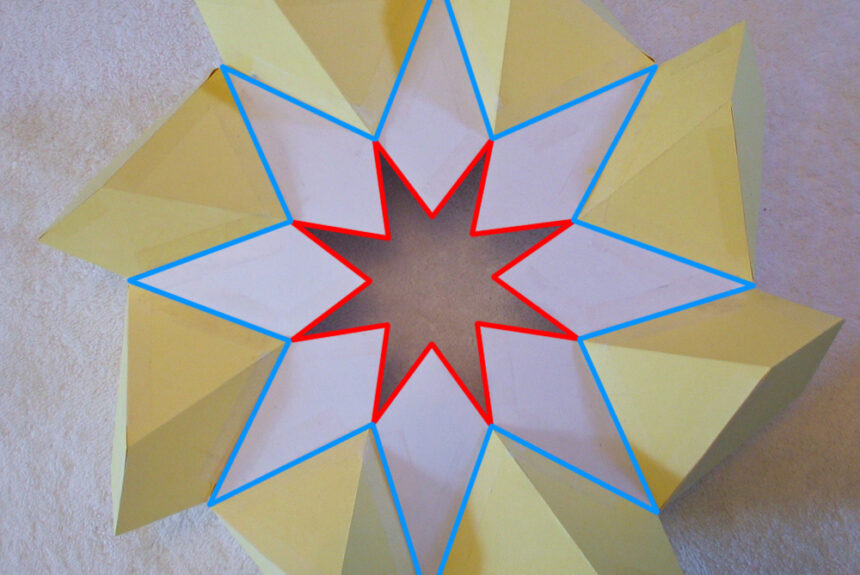
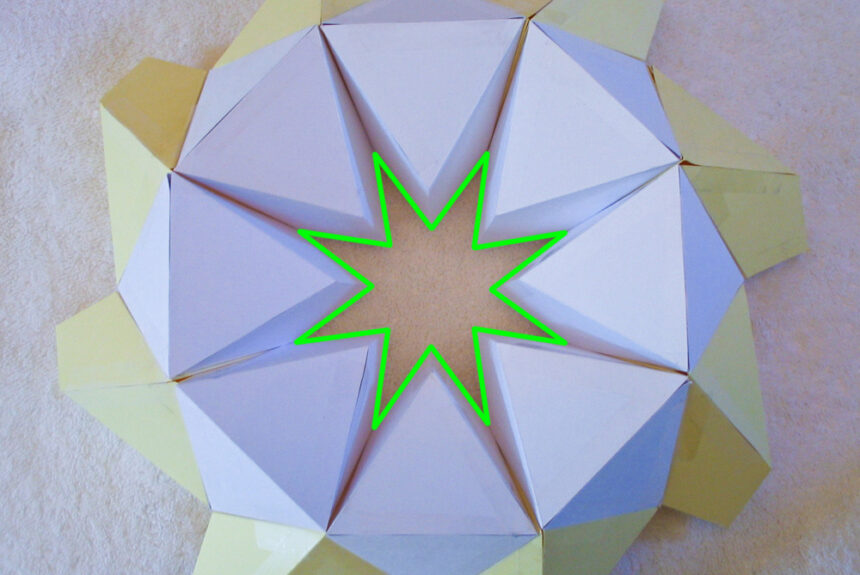
I have not (yet) made the obvious third ring, let alone a full chestahedral sphere. This involves a lot of paper, tape, and time. However, I did discover some fascinating new forms… I had wanted to see if it was possible to create a seven-fold star from the chestahedron. I tried multiple configurations but none seemed to work. But I did happen to discover an eight-fold star:

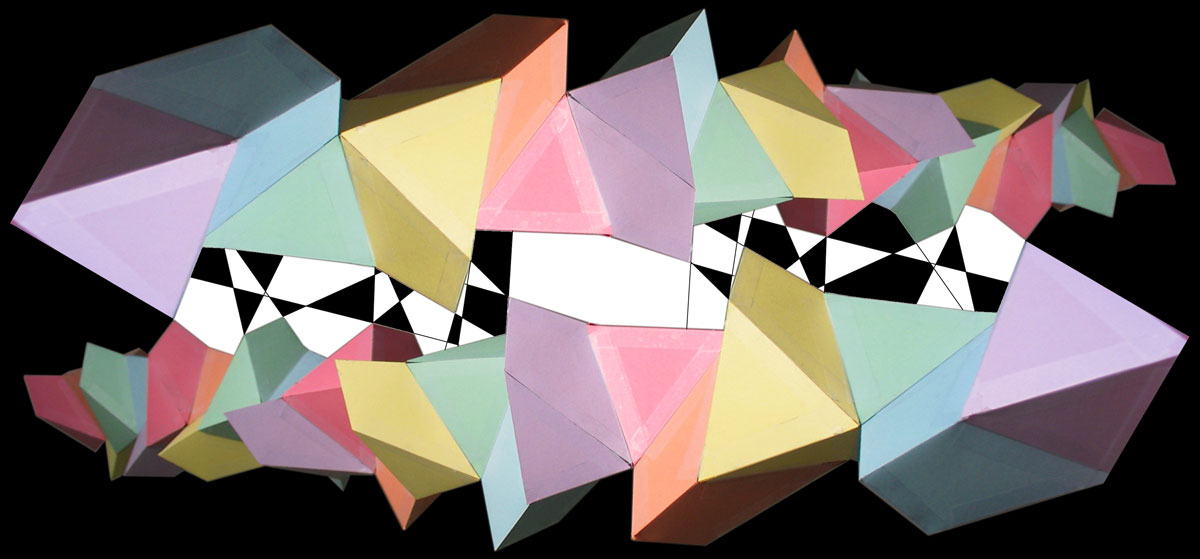
Notice the elegant eight-fold symmetry. The white kite-shaped faces do not lie in a plane, but form a subtly convex curve. Everything seems to meet perfectly. The white star has a sense of calm symmetry, while the eight yellow chestahedra seem as if they are being flung off spirally towards the periphery. The whole shape is also reminiscent of a gear or ratcheting system, even the teeth of a rotating saw blade.

When flipped over, the form is still striking. The white chestahedra form a perfect octagon, and are held apart from each other, touching only along one edge, by the yellow chestahedra, as if pulled by them. The eight most visible white triangular faces seen here form a distinct bowl shape.

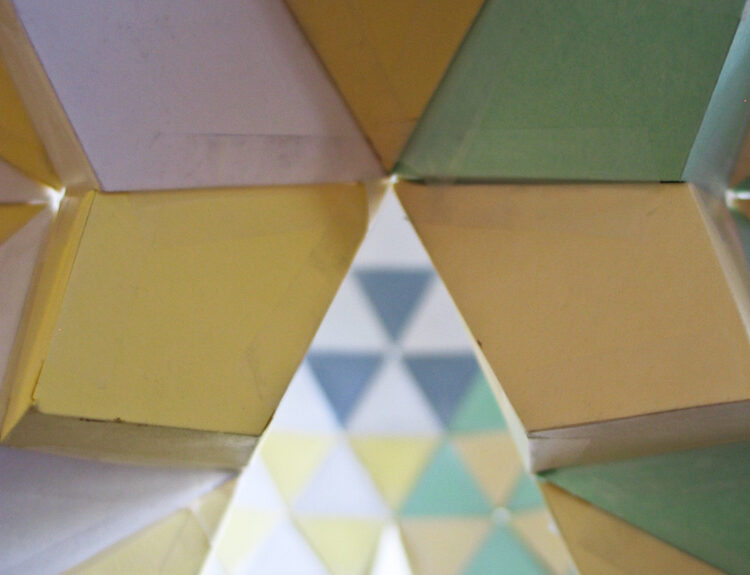
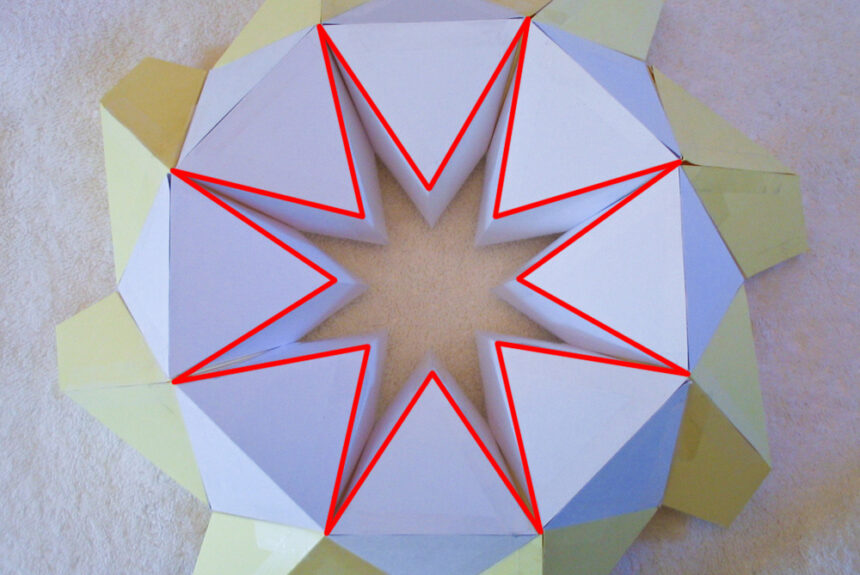
These final two views with a different background have a different flavor; the inner, negative space is emphasized, revealing eight pointed stars… more about the stars later.

It is fascinating that the chestahedron, with its seven faces of equal area, yields an eight-fold symmetry in three dimensions. This is not random by any stretch, as continued exploration shows revealing a very simple way of orienting eight chestahedra together:

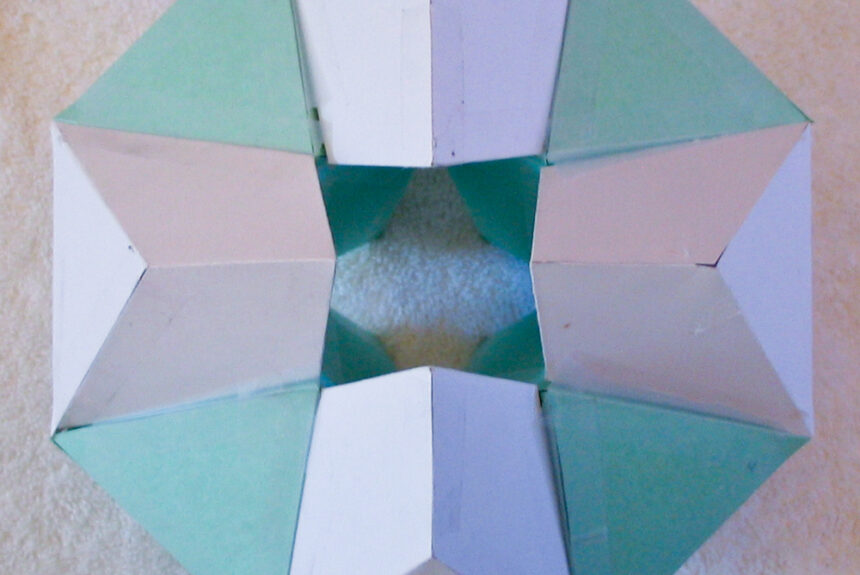
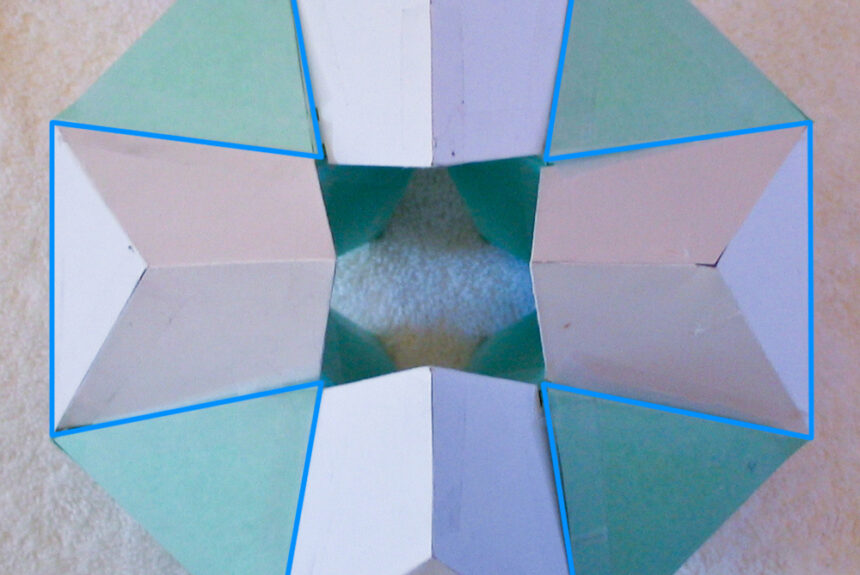
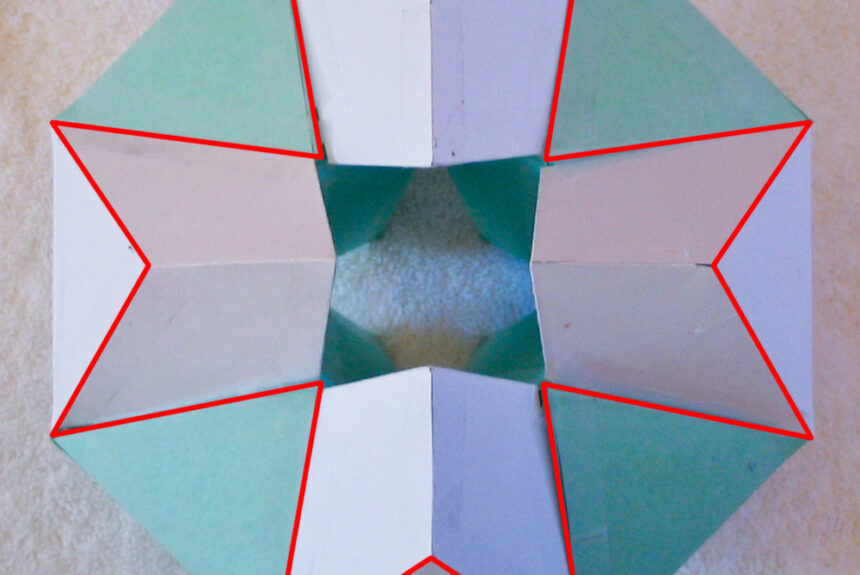
This form is made of two identically oriented sets of four chestahedra inverted with respect to each other. Take one set of four and invert it front to back, as in a mirror, and you get the other set of four. They fit together perfectly to create a double, inverted cross.

The side view shows how the chestahedra are fit together. The octagonal perimeter of the chestahedra is not twisted, but lies in a plane. The inside space of this form consists of eight kite-shaped faces alternating directions, in a tube-like or ovoid shape.

I am not aware of any types of crosses that are formed through the use of a three-dimensional form, let alone in a way that the form itself comes about as a mirror image of itself. The two cross shapes are a necessary result of the simple way in which the chestahedra are placed together; what a fascinating form to yield so many interesting shapes!

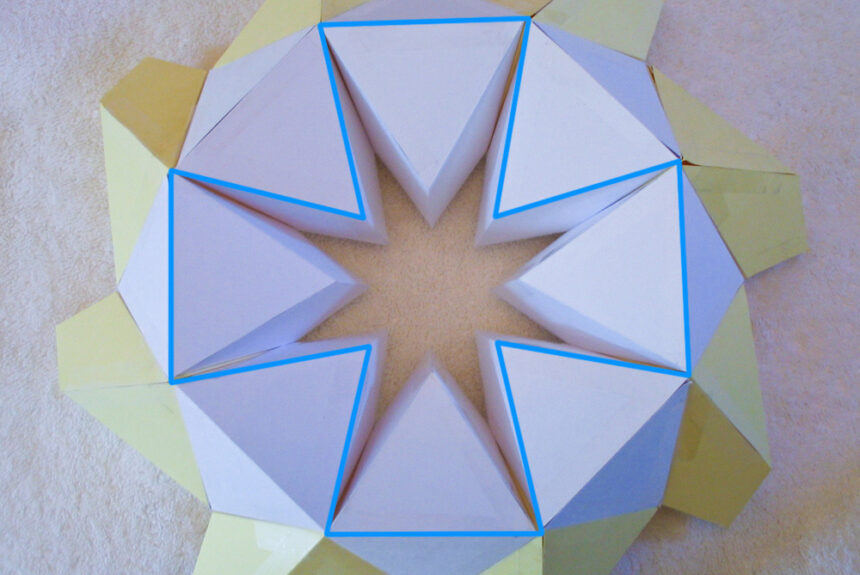
The cross, of course, has an extensive history, and there are a huge variety of forms, but a little research turned up some interesting tidbits…  The general form of the cross, where the arms flare out from the center, is known as a cross pattée. In this case the chestahedral cross is a “wedge” version of this. There are many historical variants. However, it seems that the chestahedral cross is most kin to two different versions of the cross pattée: one is a version of the Cross of St. George, the other is the Maltese cross.

This cross, with the wide inner width and flat outer feet, is used in the Swedish Rite of Freemasonry in Sweden and Scandinavia. It is a version of the St. George’s cross, which usually has non-triangular arms:

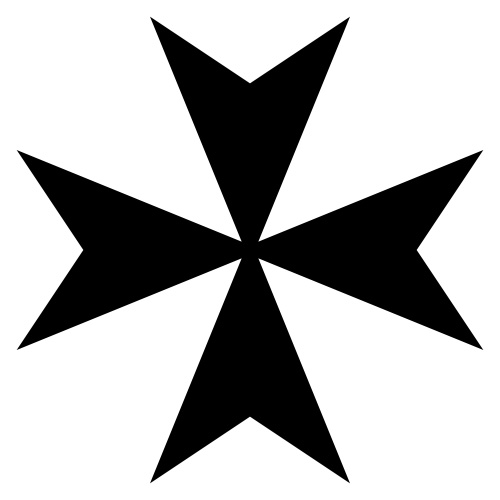
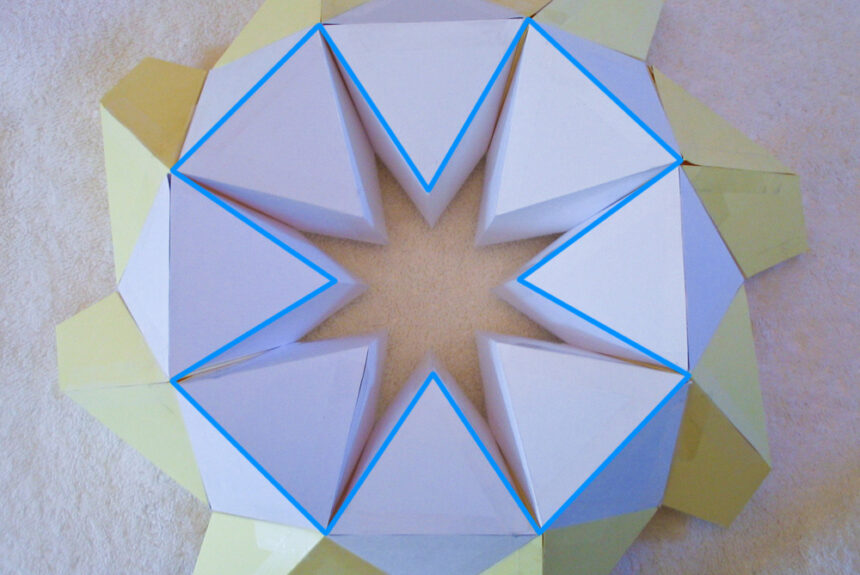
The other cross is the Maltese cross:

This cross requires noticing the three-dimensionality of the form. The Maltese cross, with its eight points, is a symbol of an order of Christian warriors, the Knights of Malta, or the Knights Hospitaller, and has a “V” shape to the arms. This group was originally founded around 1023 to provide care for poor, sick, or injured pilgrims to the Holy Land, and later became a religious and military order under its own charter, charged with the defense of the Holy Land.

So the chestahedral cross has two Maltese crosses and two crosses of St. George. What was fascinating to discover is that these same forms show up also in the chestahedral star:

Who would have thought that all this would come from the chestahedron! The many equilateral triangles on each form allow for many possible configurations… A simple rotating protocol yields a vertical column made of chestahedra in a spiral rotation very reminiscent of a DNA spiral. It is interesting that the form is capable of producing a straight column in 3-space.