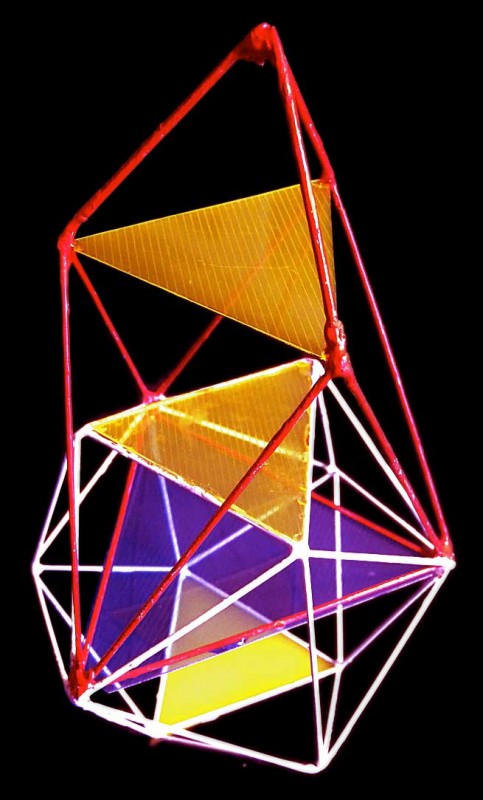
The chestahedron relates to the various Platonic forms in surprising ways. Take, for instance, the icosahedron. In the images below (click images for larger versions), you can see that the triangle faces of the icosahedron (the two bottom yellow triangles) are the same size as the triangle formed between the three points of the chestahedron (red form), as indicated by the upper yellow triangle.

This is nothing special in itself, as any single scale congruency like this can be found in isolation. However, finding an additional simultaneous scale congruency is non-trivial, and indicates a deep relationship between the chestahedron and the icosahedron. In this case, the second congruency is shown by the blue triangle, which forms the base of the chestahedron as well as an exact cross section of the icosahedron.

To help indicate this in another way, imagine the chestahedron unfolding out of a tetrahedron as in this animation. Start with the base (blue in the images below) embedded as pictured inside the static icosahedron. Now, as the tetrahedron unfolds, what is pictured below as the top-most yellow triangle starts very small (actually as the top point of the tetrahedron), and slowly grows in size. Here is the interesting thing: the moment when the unfolding of the tetrahedron reaches the exact point of creating the chestahedron, the top-most yellow triangle reaches the exact same size as one face of the icosahedron. In other words, ratios which apply to the internal structure of the icosahedron also apply to the internal structure of the chestahedron; the forms are intimately related.